AB INITIO
| Back |
|---|
As we approach the festive season of Christmas crackers, games and puzzles, it was thought once again that it would be appropriate to divert to something “Scientific” rather than strictly “Amateur Radio”. Everybody knows that the tides on the sea are due to the “pull of the moon”, but a lot of people are puzzled by a couple of aspects of this.
a) Why does a high tide also occur on the opposite side of the earth to the moon, and
b) Why is the moon gradually getting further away from us? (As astronomers tell us).
Some Answers
The answer to the first question is fairly easy. The high tide under the moon is what you would expect from gravitational attraction. The high tide on the side of the earth away from the moon is because the moon does not “go round the earth” as is commonly thought. They both go round their common centre of gravity, (sometimes and more properly called the centre of mass). The situation is represented in figure 1. Imagine a chain shot consisting of two balls of unequal weight being fired from a cannon. As they fly through the air, the larger one does not fly ballistically on a parabolic path with the smaller ball going round it. It is their common centre of gravity which travels on a ballistic path and they both fly round that. This point is marked ‘X’ in figure 1. If the chain were replaced by a rigid bar, point ‘X’ would be the point of balance. If one mass is much larger then the other, this point could be inside the larger mass.
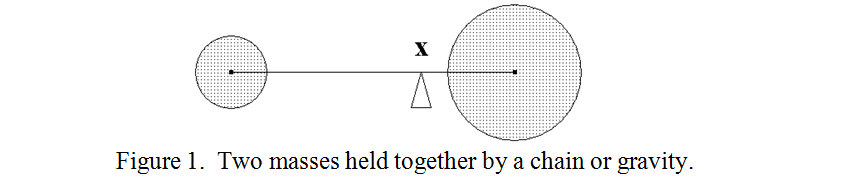
Whatever the mechanism for holding the two masses together, (a chain or gravitational attraction), both masses will be subject to “centrifugal force”. (The purists refer to this force as centripetal force, but let’s not get into that!) Centrifugal force keeps the chain tight, so that if it is broken as the masses spin, they fly apart. i.e. the force on each mass is outwards. As applied to the solid earth and the moon, this tendency to fly apart is exactly counteracted by the gravitational attraction between them. However, our fluid sea can move independently of the solid earth confining it to the orbit, and it suffers an outward force, which accounts for the high tide on the opposite side of the earth to the moon. It can be shown mathematically that the high tide under the moon is of the same magnitude as the high tide on the other side of the earth. As a matter of fact, the ‘solid’ earth also bulges a foot or two. In addition, the gravitational attraction of the sun sometimes adds and sometimes subtracts from that of the moon, leading to ‘spring’ tides and ‘neap’ tides but let’s not worry about that, it gets quite complicated.
The answer to the second question is more difficult, and employs the principle of “conservation of momentum”. (Linear momentum is simply the mass of the body times its speed. Angular momentum is the mass of the body times its rotation rate around some point or axis.) Stated simply this principle says; momentum, (both linear and angular), can neither be created nor destroyed. For example, every time you drive your car from stationary to moving, your tyres exert a force on the road which moves the whole earth in the opposite direction. The reason that you don’t notice the earth moving is because the earth is so much more massive than your car.
The moon getting further from the earth seems counter-intuitive, but it has been going on since the earth and the moon were formed some four billion years ago. It is counter intuitive because earth satellites gradually spiral in towards the earth and finally burn up. In fact you have to impart more energy by rocket power to move a satellite into a higher orbit, so where does this extra energy, (and angular momentum), come from? It comes from the tides on the sea. The mathematicians’ approach is fairly straight forward. “The friction of the tides on the rotating earth slows down the rotation of the earth and reduces its angular momentum. To compensate for this, the angular momentum of the moon has to increase, which implies a higher orbit at a slower speed”. (As a matter of fact, in the last 400 million years, the earth has slowed from 410 days in a year, (representing a 21.4 hour day), to the current 365¼ day year and 24 hour day. The current ‘slowing rate’ is a handful of milliseconds per year). The above “mathematician’s” explanation lacks detail for many people and jumps to the end result without examining the mechanism. How can angular momentum be transferred across the vacuum of space? Let us examine this.
Recap on Earth Satellites
A three stage process is involved in increasing the height of a satellite. In the first stage, the satellite in a stable orbit receives an increase in speed, (typically from a rocket engine). This causes it to move out of its original orbit at the increased speed towards a higher orbit. As it gains altitude, it looses speed, (i.e. it exchanges kinetic energy for increased potential energy), and eventually settles into the higher orbit with reduced speed. The same basic mechanism is involved in increasing the distance from the earth to the moon. The mechanism for imparting extra speed to the moon by the earth’s tides can be explained as follows.
More detailed explanation
Looking down from space onto the North pole of the earth, the relative positions and motions of the earth, its oceans and the moon might be thought of as shown in figure 2. (London may be imagined to be where the upper label for “Low tide” is and New York where the right hand label for “High Tide” is. Hence London sees the dawn before New York does.
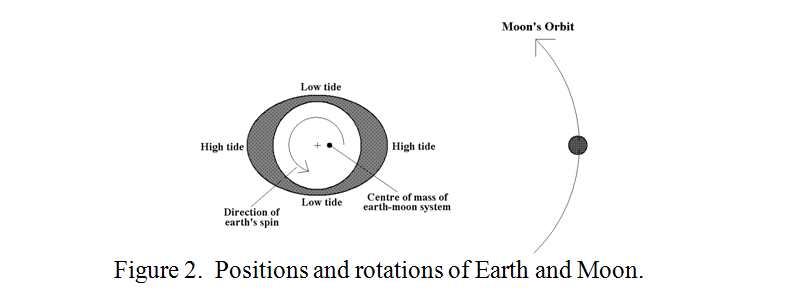
However, figure 2 represents a static situation. The earth spins at a relatively rapid rate compared with the orbital rate of the moon, so that we can imagine the moon to be stationary compared to the earth. As the earth spins, (at about 1000mph at the equator), it takes its seas with it, so that the seas are rushing past the line of maximum pull of the moon. The “drag” of the spinning earth on the sea results in a “phase shift” of the position of high tide from directly “under” the moon to more like that shown in figure 3.
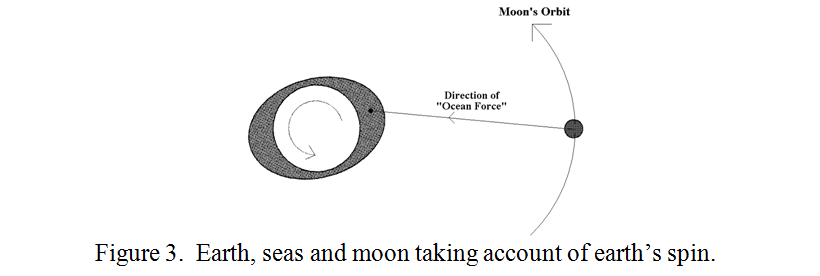
This also accounts for another puzzling fact: that a high tide is experienced after the moon has passed overhead. The high tide under the moon has a greater effect on the moon than the high tide on the far side of the earth on account of its proximity. This gravitational force, (due to the nearest high tide), is relatively small compared with the forces keeping the moon in orbit but it is a force not entirely along the line joining the centres of the earth and moon, as indicated by the “Ocean Force” line of figure 3. This force has a component in the direction tending to accelerate the moon in its orbit, giving it more energy. This, just like a satellite being pushed into a higher orbit, eventually leads to a higher orbit at a lower speed for the moon.
| Back |
|---|
PRAECEPTOR